Quenching of Orbital Angular Momentum

Orbital angular momentum arises due to the rotation of orbitals. It has been found that orbital angular momentum is partially reduced even in fee ion and in crystal field.
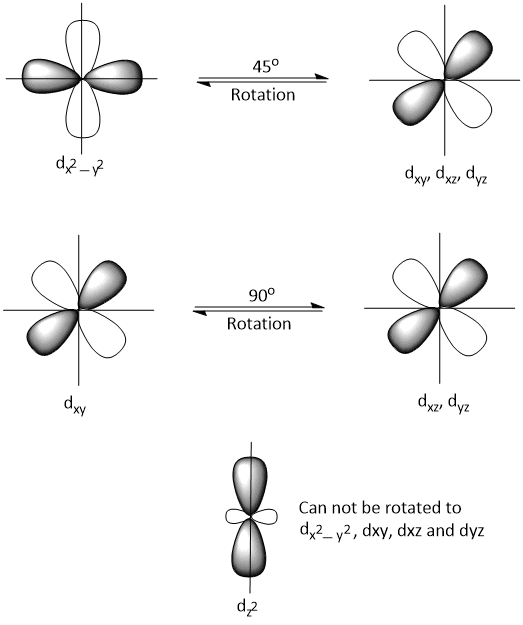
In free ion, d_xy, d_xz and d_yz orbitals can rotate by 90° to each other and d_x²−y² can not be rotated by 45° to d_xy, d_xz and d_yz and vice-versa but d_z² can not be rotated to d_x²−y² or d_xy, d_xz and d_yz. Thus, one fifth of the orbital angular momentum is reduced or quenched even in free ion.

In the crystal field, d-orbital splits as-

The energy of d_x²−y² and d_xy, d_xz and d_yz orbitals are differ in crystal field and so, d_x²−y² can not be rotated to d_xy, d_xz and d_yz and vice-versa to give orbital of equivalent energy. Hence, in the crystal field, there is a further reduction or quenching of orbital angular momentum.
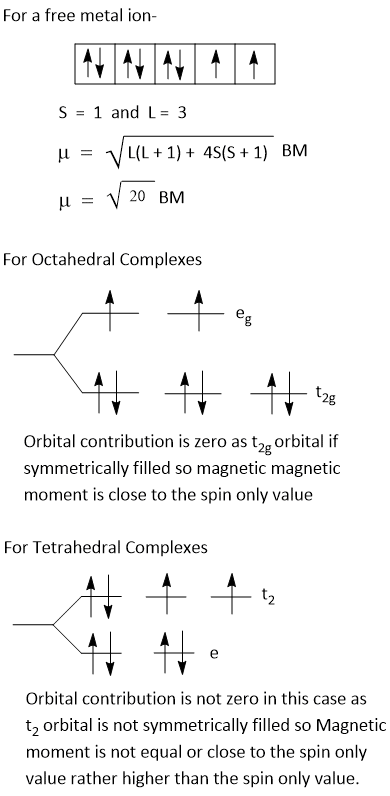
Orbital contribution to magnetic moment is only due to t_2g or t₂ rotation. However, if t_2g or t₂ are evenly occupied by electrons, as t_2g³, t_2g⁶, t₂³, t₂⁶, then orbital contribution to magnetic moment is fully quenched and in that case μ_eff-

Orbital contribution occurs only for t_2g¹e_g^x, t_2g²e_g^x, t_2g⁴e_g^x, t_2g⁵e_g^x or for corresponding t₂.
If ground term in crystal field is T, due to orbital contribution μ_eff is greater than μ_s. Thus, for d¹, d⁴, d⁶, d⁷ weak field octahedral crystal field , d³, d⁴, d⁸, d⁹ in tetrahedral crystal field, d¹, d², d⁴, d⁵ in strong octahedral crystal field, there is orbital contribution and magnetic moment of these is greter than μ_s value.

Let's Consider a Ni(II) complex-
Electronic configuration is d⁸

Why orbital angular momentum is not quenched in 4d and 5d elements?

 Share